
In fact, every element can be a left identity. It demonstrates the possibility for ( S, ∗) to have several left identities.
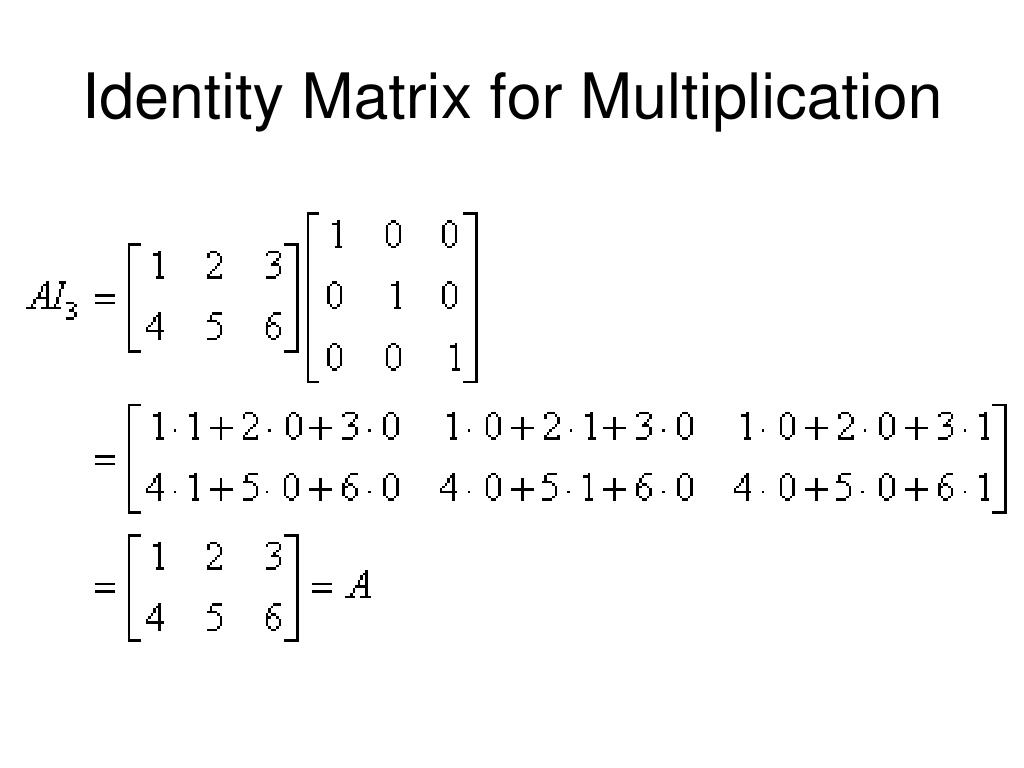
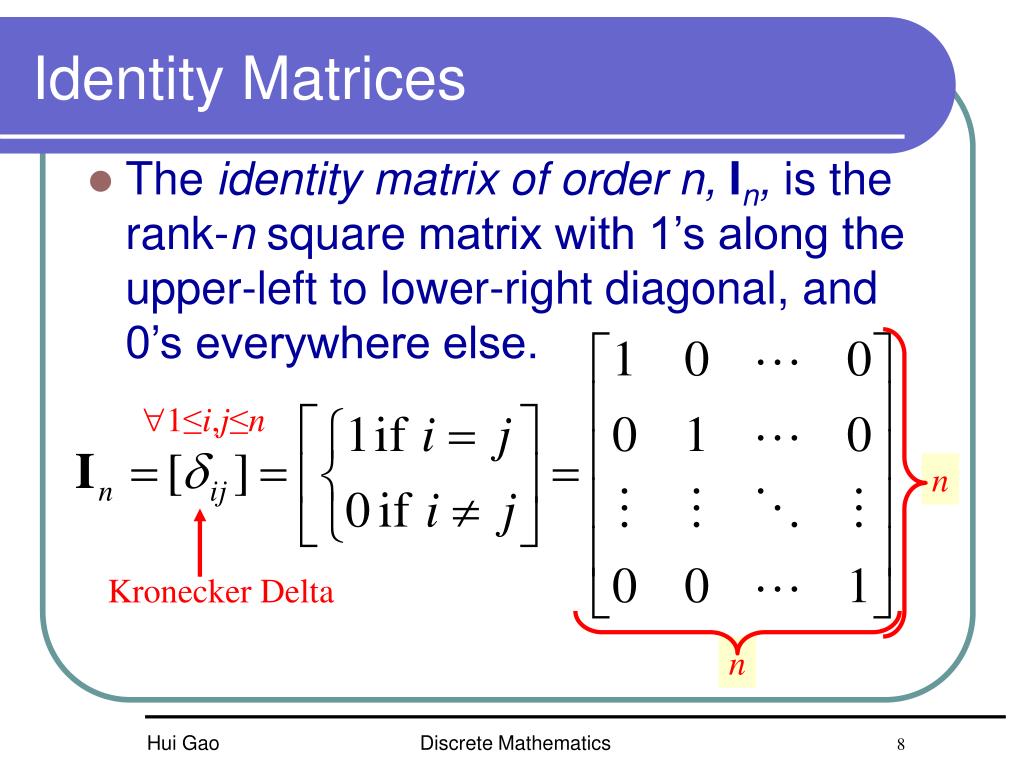
In the case of a group for example, the identity element is sometimes simply denoted by the symbol e with the equalities given, S is a semigroup. These need not be ordinary addition and multiplication-as the underlying operation could be rather arbitrary. Īn identity with respect to addition is called an additive identity (often denoted as 0) and an identity with respect to multiplication is called a multiplicative identity (often denoted as 1). If e is both a left identity and a right identity, then it is called a two-sided identity, or simply an identity. Then an element e of S is called a left identity if e ∗ s = s for all s in S, and a right identity if s ∗ e = s for all s in S. Let ( S, ∗) be a set S equipped with a binary operation ∗. The term identity element is often shortened to identity (as in the case of additive identity and multiplicative identity) when there is no possibility of confusion, but the identity implicitly depends on the binary operation it is associated with. This concept is used in algebraic structures such as groups and rings. In mathematics, an identity element, or neutral element, of a binary operation operating on a set is an element of the set that leaves unchanged every element of the set when the operation is applied. Specific element of an algebraic structure


 0 kommentar(er)
0 kommentar(er)
